Four Thousand Years Ago in Egypt...

If you had been a priest in ancient Egypt, you would have been in the highest class of men. You would have had great power and control over mere mortals and held great sway with the Pharoah. You would have been knowledgeable in many subjects, not the least of which was mathematics. Your ability to reckon and keep records would have made you indispensible. And you would have jealously guarded this secret power, teaching it only to a select few who would work for you and later, perhaps, take your place.

But your ability to reckon would have been nothing like ours. In fact, the numbers you used would have looked completely different. Not only would you have used different symbols, but you would have formed large numbers in a manner much like the Romans would use. You would have used an additive numeration system and thus your techniques for adding and subtracting would have differed vastly from our algorithms.

The Eyptians had only a few symbols with which to create numbers.

The first symbol is called a stroke and is worth just 1, a unit. The second symbol is the heel bone and is worth 10 strokes. The third symbol is the scroll, worth ten heel bones, or 100 strokes. The fourth is a lotus flower and is worth ten scrolls, or 1000 strokes. Fifth is the pointing finger and is worth ten lotus flowers or 10,000 strokes. Sixth is the tadpole, worth ten pointing fingers or 100,000 strokes. And finally is the astonished man, worth ten tadpoles or one million strokes.

To create a number, you add together as many of each symbol as necessary to form the value. For instance, to create the Egyptian number that represents 47, you would need four heel bones and seven strokes. It doesn't really matter which order you put the symbols in, but by convention, the symbols were written in groups ordered from smallest to biggest. So this number would be shown with the seven strokes followed by the four heel bones.

In the same way, you can convert an Egyptian number to our system by adding the values of the symbols together. For example:

Counting the amount of each symbol, we see six strokes, five heel bones, and three scrolls. That makes six 1's, five 10's, and three 100's. In our number system, we'd write that as 356 or three hundred fifty six.

Suggested Activities:

1. Write the following in Egyptian numbers: your age; your weight; the year of your birth; the cost of a new Jaguar car (any model); the current population of the state in which you live; the current population of the world.
2. See if you can find a way to add two Egyptian numbers together, such as 839 and 427. Don't convert them into our numbers first and then switch back to Egyptian. Think like an Egyptian and use only their symbols. You might discover why we "carry" when we're adding numbers together.
3. See if you can find a way to subtract two Egyptian numbers, such as 5493 and 3586. Again, only use Egyptian symbols and don't convert into our number system. You just might discover what "borrowing" really means.
4. Write a short essay explaining what happens when you try to write numbers such as 200 or 40,008.
5. Find a foolproof method for determining the total number of symbols required to write any number in Egyptian. For example, how many symbols do you need to write the number 2004? 24,489? 9,999,999? Is there a general method you can use that works ALL the time?
6. Take a look at this image of the Moscow Papyrus, one of the few Ancient Egyptian mathematical texts in existence. Can you see any numbers?

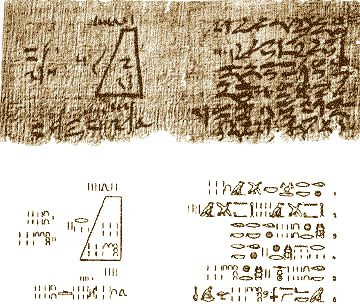
I'll post an article on addition and subtraction next so that you can check your method against the Egyptian way.